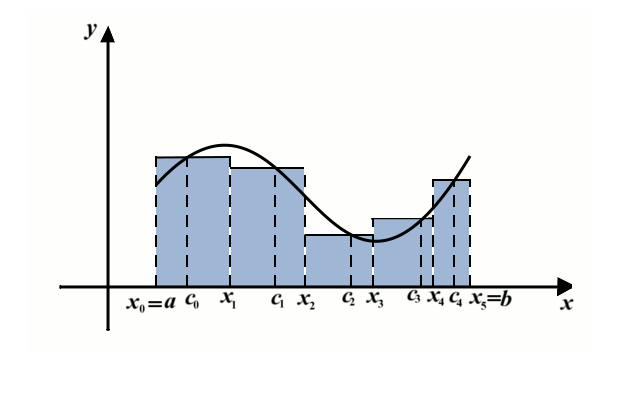Definite Integrals. Fundamental Theorem of Calculus
Integral Sum
The definite integral can be understood as the area under the graph of the function. In order to define the integral properly, we need the concept of integral sum.
Assume that \(f\) is a continuous function defined on the interval \([a,b]\). A partition of the interval \([a,b]\) is a sequence of points \(x_0\), \(x_1\), \(\dots\), \(x_n\) such that \(x_0=a\), \(x_n=b\), and \(x_0\leq x_1\leq x_2\leq\cdots\leq x_n\).
Let \(c_0\) be any point from the interval \([x_0,x_1]\), \(c_1\in[x_1,x_2]\), \(\dots\), \(c_{n-1}\in [x_{n-1},x_n]\). An integral sum (also called the Riemann sum) corresponding to the partition \(x_0\), \(x_1\), \(\dots\), \(x_n\), and the numbers \(c_0\), \(c_1\), \(\dots\), \(c_{n-1}\) is defined as \[S(f,x_0,x_1,\dots, x_n,c_0,\dots, c_{n-1})=f(c_0)\cdot (x_1-x_0)+f(c_1)\cdot (x_2-x_1)+f(c_2)\cdot (x_3-x_2)+\cdots+ f(c_{n-1})\cdot (x_n-x_{n-1}).\]
This quantity \(S\) depends on the choice \(c_0\), \(c_1\), \(\dots\), \(c_{n-1}\), and the partition \(x_0\), \(x_1\), \(\dots\), \(x_n\). The surface area of the shaded region in the picture below is an example for the integral sum with \(n=5\).
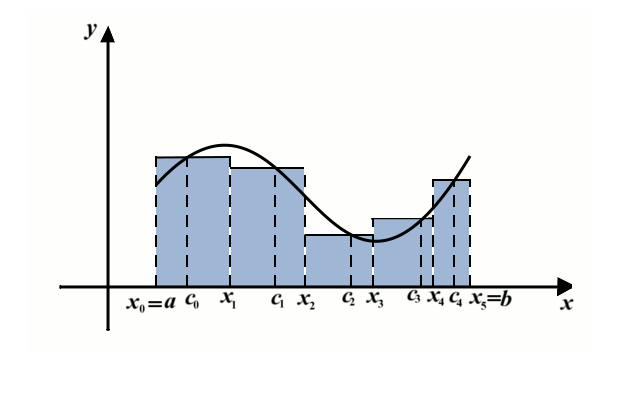
The maximum of the numbers \(\{|x_1-x_0|, |x_2-x_1|,\dots, |x_n-x_{n-1}|\}\), usually denoted by \(\delta\) is called the diameter of the partition. The limit of the integral sums as the diameter of the partition approaches \(0\) is called the integral of the function \(f\) on the interval \([a,b]\) and is denoted by \(\int_a^b f(x)\,dx\). Here is the precise definition:
Definition (Definite integral)
A function \(f:[a,b]\to\mathbb R\) is integrable and its integral over the segment \([a,b]\) is equal to a real number \(I\in\mathbb R\) if for each \(\varepsilon > 0\) there exists \(\delta > 0\) such that for every partition \(a=x_0\leq x_1\leq\dots\leq x_n=b\) with \(\delta(x_0,\dots, x_n) < \delta\) we have \[|S(f,x_0,x_1,\dots, x_n,c_0,\dots, c_n)-I| < \varepsilon\] for every choice of \(c_0\in[x_0,x_1]\), \(c_1\in[x_1,x_2]\), \(\cdots\), \(c_{n-1}\in[x_{n-1},x_n]\).
The above-described limit does not exist for all functions \(f\), but if such a limit exists, the function is called integrable/ All continuous functions are integrable. Also, all bounded functions that have countably many discontinuities are integrable as well. The class of integrable functions is surprisingly large, but our aim is not to go into details at this point.
Fundamental theorem of calculus
The fundamental theorem of calculus makes a connection between antiderivatives and definite integrals. The first theorem that we will present shows that the definite integral \(\int_a^xf(t)\,dt\) is the anti-derivative of a continuous function \(f\). This theorem is sometimes referred to as First fundamental theorem of calculus. Its name is actually differentiation theorem.
Theorem (Differentiation Theorem)
Assume that \(f\) is a continuous function on \([a,b]\). Let \(x\in(a,b)\), and let \(F(x)=\int_a^xf(t)\,dt\). Then for each \(x\in(a,b)\), \(F\) is a differentiable function and \(x\in(a,b)\) and \begin{eqnarray*}F’(x)=f(x).\end{eqnarray*}
We will prove that for every \(\varepsilon > 0\) there exists \(\delta\) such that for each \(y\in(x-\delta, x+\delta)\) we have \[\frac{F(y)-F(x)}{y-x}\in\left(f(x)-\varepsilon,f(x)+\varepsilon\right). \quad\quad\quad\quad\quad (1)\]
Assume that \(x\in(a,b)\) and \(\varepsilon > 0\) are given. Let \(\delta_1=\frac12\min\{x-a,b-x\}\) and let \(\delta_2 > 0\) be a real number such that \(y\in [x-\delta_2,x+\delta_2]\) implies \(|f(x)-f(y)| < \varepsilon\). The existence of \(\delta_2\) follows from the continuity of \(f\). Let \(\delta=\min\{\delta_1,\delta_2\}\).
We will now prove that for each \(y\in(x-\delta, x+\delta)\) the inequality (1) is satisfied. Assume that \(y\in(x,x+\delta)\). The case \(y\in(x-\delta, x)\) is analogous. Let us denote by \(m\) and \(M\) the minimum and the maximum of \(f\) in the interval \([x,y]\). The continuity of \(f\) implies the existence of these two numbers as well as \([m,M]\subseteq \left(f(x)-\varepsilon, f(x)+\varepsilon\right)\). Since \(m\leq f(x)\leq M\) on \([x,y]\) we have:
\[m(y-x)\leq \int_x^y f(t)\,dt\leq M(y-x).\]
Notice that \(F(y)-F(x)=\int_x^yf(t)\,dt\) hence \[ \frac{F(y)-F(x)}{y-x}\in[m,M]\subseteq \left(f(x)-\varepsilon,f(x)+\varepsilon\right).\]
This completes the proof of the theorem.
Here we will present the formulation and the proof of the fundamental theorem of calculus. This theorem is also known as Newton-Leibnitz formula.
Theorem (Fundamental Theorem of Calculus)
If \(f\) is a continuous function on \([a,b]\) and \(F\) its antiderivative, then \[\int_a^b f(x)\,dx=F(b)-F(a).\]
Remark.
There are many generalizations of this theorem that can relax our assumptions of continuity of \(f\).
Let \(\delta > 0\) and let \((x_i)_{i=0}^n\) be an increasing sequence of numbers from the interval \((a,b)\) such that \(x_0=a\), \(x_n=b\), and \(|x_{i+1}-x_i|\leq \delta\) for all \(i\in\{0,1,\dots, n-1\}\).
According to the mean-value theorem, there exists a sequence of real numbers \((\xi_i)_{i=0}^{n-1}\) such that \(\xi_i\in(x_i,x_{i+1})\) and \[\frac{F(x_{i+1})-F(x_i)}{x_{i+1}-x_i}=F^{\prime}(\xi_i).\] The following equality holds: \[F(b)-F(a)=\sum_{i=0}^{n-1} F(x_{i+1})-F(x_i)=\sum_{i=0}^{n-1} F^{\prime}(\xi_i)\cdot (x_{i+1}-x_i)=\sum_{i=0}^{n-1} f(\xi_i)\cdot (x_{i+1}-x_i).\]
The last sum converges to \(\int_a^b f(x)\,dx\) as \(\delta\to0\).
Examples
In evaluating the integrals \(\displaystyle \int_a^bf(x)\,dx\) one needs to find an antiderivative \(F\) of the function \(f\), and to calculate \(F(b)-F(a)\). The notation \(\displaystyle \left.F(x)\right|_{a}^b\) is often used to replace the expression \(F(b)-F(a)\). Sometimes we also use \(\displaystyle \left.F(x)\right|_{x=a}^{x=b}\) for the same purpose especially if the function \(F\) depends on some other parameters and we want to avoid any possible ambiguity.
Example 1.
Evaluate the integral \(\displaystyle \int_0^6x^2\,dx\).
Since \(F(x)=\frac{x^3}3\) satisfies \(F^{\prime}(x)=x^2\) we have that \[ \int_0^6x^2\,dx= \left.\frac{x^3}3\right|_{x=0}^{x=6}=\frac{6^3}{3}-0=72.\]
Example 2.
Evaluate the integral \(\displaystyle \int_0^{\frac{\pi}2}\cos x\,dx\).
The antiderivative of \(\cos x\) is \(\sin x\). More precisely, \(\left(\sin x\right)^{\prime}=\cos x\) hence:
\[\int_0^{\frac{\pi}2}\cos x\,dx=\left.\sin x\right|_0^{\frac{\pi}2}=\sin\frac{\pi}2-\sin 0=1.\]
Example 3.
Evaluate the integral \(\displaystyle \int_1^e\ln x\,dx\).
Notice that the function \(F(x)=x\ln x-x\) satisfies \(F^{\prime}(x)=\ln x\). Therefore \[\int_1^e\ln x\,dx=\left. \left(x\ln x-x\right)\right|_1^e=e\ln e-e-1\ln1+1=
e-e-0+1=1.\]
Remark. We found the anti-derivative of \(\ln x\) using guessing. Once we cover the integration by parts we will see that there is a method for calculating the anti-derivative of \(\ln x\) that does not involve magic. Guessing will always remain an option, but one should try to learn more dependable methods.
Practice problems
Problem 1. Find the integral \[\int_3^9 \left(x^2-3\right)\,dx.\]
According to the Fundamental Theorem of Calculus, we have \[\int_3^9 \left(x^2-3\right)\,dx=F(9)-F(3),\]
where \(F\) is an anti-derivative of the function \(x^2-3\). We can find \(F\) in the following way:
\[\int\left(x^2-3\right)\,dx=\int x^2\,dx-\int 3\,dx=\frac13x^3-3x+C.\]
Since \(F\) can be any anti-derivative we may choose \(C=0\), and we get \[\int_3^9\left(x^2-3\right)\,dx=\left(\frac13\cdot 9^3-3\cdot 9\right)-\left(\frac13 \cdot 3^3-3\cdot 3\right)=216.\]
Problem 2. Evaluate the integral \[\int_2^{8}\left(\frac1x+x^2\right)\,dx.\]
We first need to find an anti-derivative \(F(x)\) of the function \(f(x)=\frac1x+x^2\), and, according to the Fundamental Theorem of Calculus, the given area is equal to \(F(8)-F(2)\). Using indefinite integrals to denote the anti-derivatives we obtain:
\begin{eqnarray*}
\int \left(\frac1x+x^2\right)\,dx&=&\int \frac1x\,dx+\int x^2\,dx=\ln |x|+\frac{x^3}3+C.
\end{eqnarray*}
We may pick any value of \(C\), and with so-obtained function \(F\) we can calculate \(F(8)-F(2)\). Let us take \(C=0\). _/p__p_
Then we have \begin{eqnarray*}\int_2^8\left(\frac1x+x^2\right)\,dx=F(8)-F(2)=\left(\ln 8+\frac{512}3\right)-\left(\ln 2+\frac83\right)=\ln \frac{8}2+\frac{504}3=\ln 4+168.\end{eqnarray*}
Problem 3. Find the area bounded by the lines \(x=1\), \(x=3\), the \(x\)-axis, and the parabola \(y=x^2+3\).
The problem is asking us to evaluate \(\int_1^3\left(x^2+3\right)\,dx\). We first need to find an anti-derivative \(F(x)\) of the function \(f(x)=x^2+3\), and, according to the Fundamental Theorem of Calculus, the given area is equal to \(F(3)-F(1)\). Using indefinite integrals to denote the anti-derivatives we obtain:
\begin{eqnarray*}
\int \left(x^2+3\right)\,dx&=&\int x^2\,dx+\int 3\,dx=\frac13x^3+3x+C.
\end{eqnarray*}
We may pick any value of \(C\), and with so-obtained function \(F\) we can calculate \(F(3)-F(1)\). Let us take \(C=0\). _/p__p_
Then we have \begin{eqnarray*}\int_1^3\left(x^2+3\right)\,dx=F(3)-F(1)=\left(\frac{27}3+9\right)-\left(\frac13+3\right)=15-\frac13=\frac{44}3.\end{eqnarray*}
Problem 4. Find the area bounded by the lines \(x=1\), \(x=5\), the \(x\)-axis, and the parabola \(y=x^2+2x+7\).
The problem is asking us to evaluate \(\int_1^5\left(x^2+2x+7\right)\,dx\). We first need to find an anti-derivative \(F(x)\) of the function \(f(x)=x^2+2x+7\), and the given area is equal to \(F(5)-F(1)\), according to the Fundamental Theorem of Calculus. Using indefinite integrals to denote the anti-derivatives we obtain:
\begin{eqnarray*}
\int \left(x^2+2x+7\right)\,dx&=&\int x^2\,dx+\int 2x\,dx+\int 7\,dx=\frac13x^3+ x^2+7x+C.
\end{eqnarray*}
We may pick any value of \(C\), and with so-obtained function \(F\) we can calculate \(F(5)-F(1)\). Let us take \(C=0\). _/p__p_
Then we have \begin{eqnarray*}\int_1^5\left(x^2+2x+7\right)\,dx=F(5)-F(1)=\frac{124}3+24+28=52+\frac{124}3=\frac{280}3.\end{eqnarray*}
Problem 5. Evaluate the integral \[\int_{-1}^1 \frac{x^2}{1+x^2}\,dx.\]
We first need to find an anti-derivative \(F(x)\) of the function \(f(x)=\frac{x^2}{x^2+1}\), and according to the Fundamental Theorem of Calculus, the given definite integral is equal to \(F(1)-F(-1)\). Using indefinite integrals to denote the anti-derivatives we obtain:
\begin{eqnarray*}
\int \frac{x^2}{1+x^2}\,dx&=&\int \frac{1+x^2}{1+x^2}\,dx-\int \frac1{1+x^2}\,dx=x-\arctan x+C.
\end{eqnarray*}
We may pick any value of \(C\), and with so-obtained function \(F\) we can calculate \(F(1)-F(-1)\). Let us take \(C=0\). _/p__p_
Then we have \begin{eqnarray*}\int_{-1}^{1}\frac{x^2}{1+x^2}\,dx=F(1)-F(-1)= \left(1-\arctan 1\right)-\left(-1-\arctan(-1)\right)=2-2\arctan 1=2-\frac{\pi}2.\end{eqnarray*}