Supply, Demand, Producer Surplus, and Consumer Surplus
We will start by analyzing one example of a very simplified market. Assume that we own a business called Souvenir Shop that Doesn't Make Too Much Money. Assume that we are selling only one kind of souvenir: a statue of a frog. We need to obtain the frogs directly from the artists and then we need to sell the frogs to the tourists. Each frog will have the same price \(p\). We will pay the price \(p\) for each frog to the artist who makes it. Then we will receive \(p\) from each tourist who buys the frog. The price we pay is the same as the price we sell for. We won't make any money in the process, and hence the name of the store.

In this article we will analyze how different decisions on price \(p\) can affect our artists and our tourists. This is a very basic example, so don’t start this non-money making business at home. This text will teach you basic concepts in economics and math. Then you will have to read something else to learn more advanced and useful theories on how to make (and lose) money.
There are 7 artists that we can collaborate with: Salvador Dali, Vincent van Gogh, Leonardo da Vinci, Kazimir Malevich, Michelangelo Buonarroti, Pablo Picasso, and Jackson Pollock. Each has offered to make one frog for our souvenir shop. We assume that each of them is qualified to make a frog, all of them are able to make exactly the same looking frog, and none of them is going to put a signature or some weird symbol on the frog to make it more attractive to certain collectors.
Supply
In this example, the artists are called suppliers. They are providing us with frogs. We are paying the suppliers to get the frogs. Suppliers are getting money to make these frogs.
We will now list the amounts of money that the artists want to charge us. The charges are listed in increasing order.
- 1. Dali can make a frog and sell it to us for \(\$2\).
- 2. van Gogh would charge us \(\$ 3\);
- 3. Leonardo asks for \(\$ 5\);
- 4. Malevich asks for \(\$ 6\);
- 5. Michelangelo asks for \(\$ 8\);
- 6. Picasso asks for \(\$9\);
- 7. Pollock asks for \(\$11\).
We will now put this data in a little bit strange graph. The prices will be on the vertical axis. The horizontal axis will be denoted by \(x\). The coordinate \(x=1\) corresponds to the cheapest supplier. In our example, it is Salvador Dali. The coordinate \(x=2\) corresponds to the next cheapest - Vincent van Gogh. The most expensive one (Jackson Pollock) will receive the number 7. The obtained picture is called the supply graph.
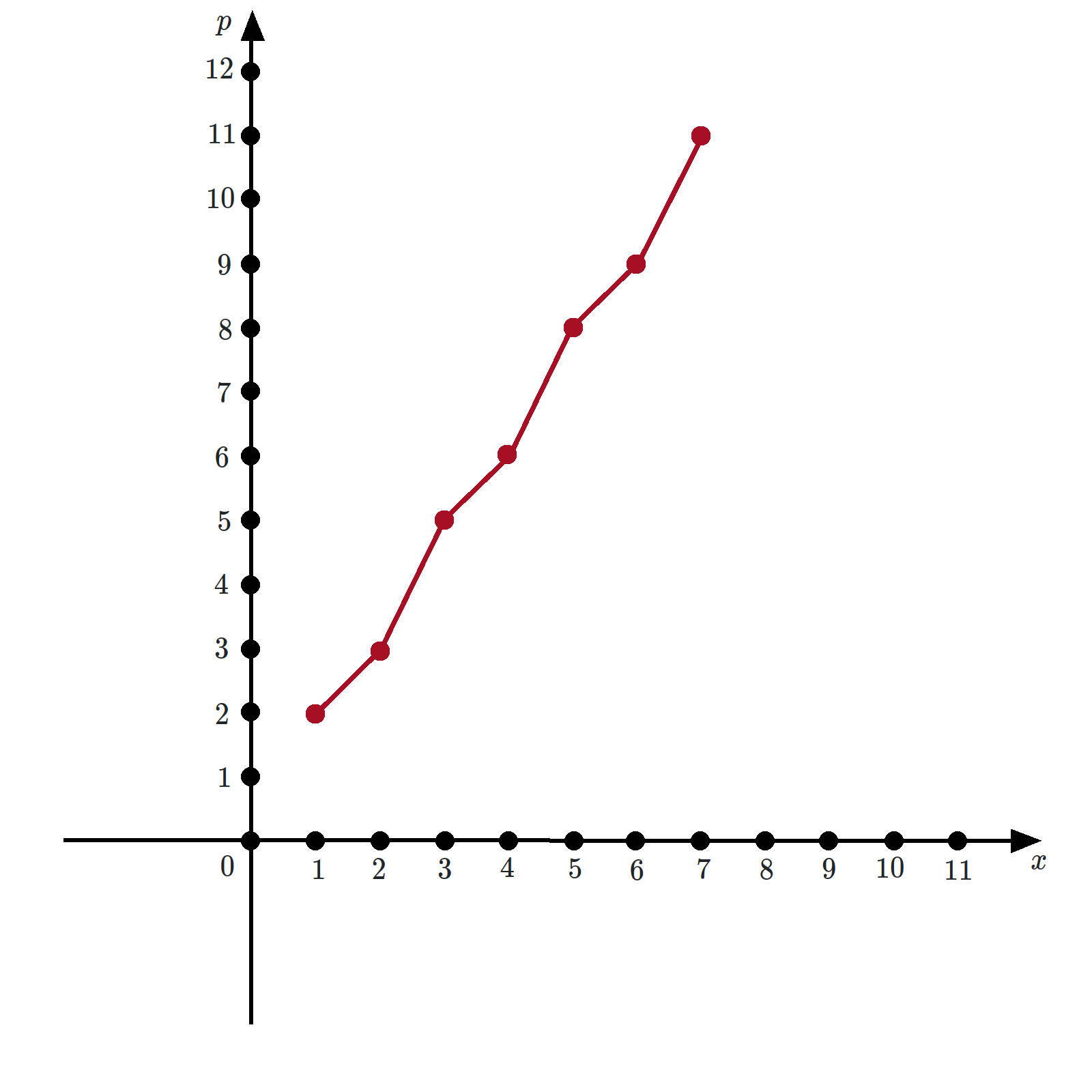
Supply is the function whose input is price \(p\) and output is the number of items that suppliers are willing to make if the price is set to \(p\).
The supply function will be denoted by \(\sigma\). For given price \(p\), we denote by \(\sigma(p)\) the total number of items that would be produced if the price is set to \(p\)
Supply function can be read from the graph. Observe that in our graph the prices are on the vertical axis and the supplies are on the horizontal axis.
In our example, the graph should only consist of individual points. However, we drew the line through the points to make the picture nicer. Later on we will consider more general situation in which the supply function is continuous (and even differentiable).
Demand
It is now time to meet our potential customers. There are only 7 of them and their names are: Julius Caesar, Genghis Khan, George Washington, Winston Churchill, Mao Zedong, Joseph Stalin, and Diego Maradona.
Each of them is ready to pay for the frog. Some of them would pay a lot, the others are more reasonable. We will list the amounts that the consumers would pay in decreasing order.
- 1. Julius Caesar would buy a frog even if its cost is \(\$11\).
- 2. Genghis Khan would buy a frog if its cost is \(\$10\) or less.
- 3. George Washington has \(\$8\) and is willing to spend all of it if necessary to buy his frog.
- 4. Winston Churchill has \(\$6\). He is ready to spend all of the money to buy a frog.
- 5. Mao Zedong would pay at most \(\$5\) for a frog.
- 6. Joseph Stalin would pay at most \(\$4\) for a frog.
- 7. Diego Maradona would pay at most \(\$2\) for a frog.
We will put this data on the graph. Again, the vertical axis will correspond to prices. We use label \(p\) for the vertical axis. The horizontal axis will be labeled by \(x\). The coordinate \(x=1\) corresponds to Julius Caesar - the consumer who is willing to pay the most. The coordinate \(x=2\) corresponds to the next consumer. The coordinate \(x=7\) corresponds to Diego Maradona.
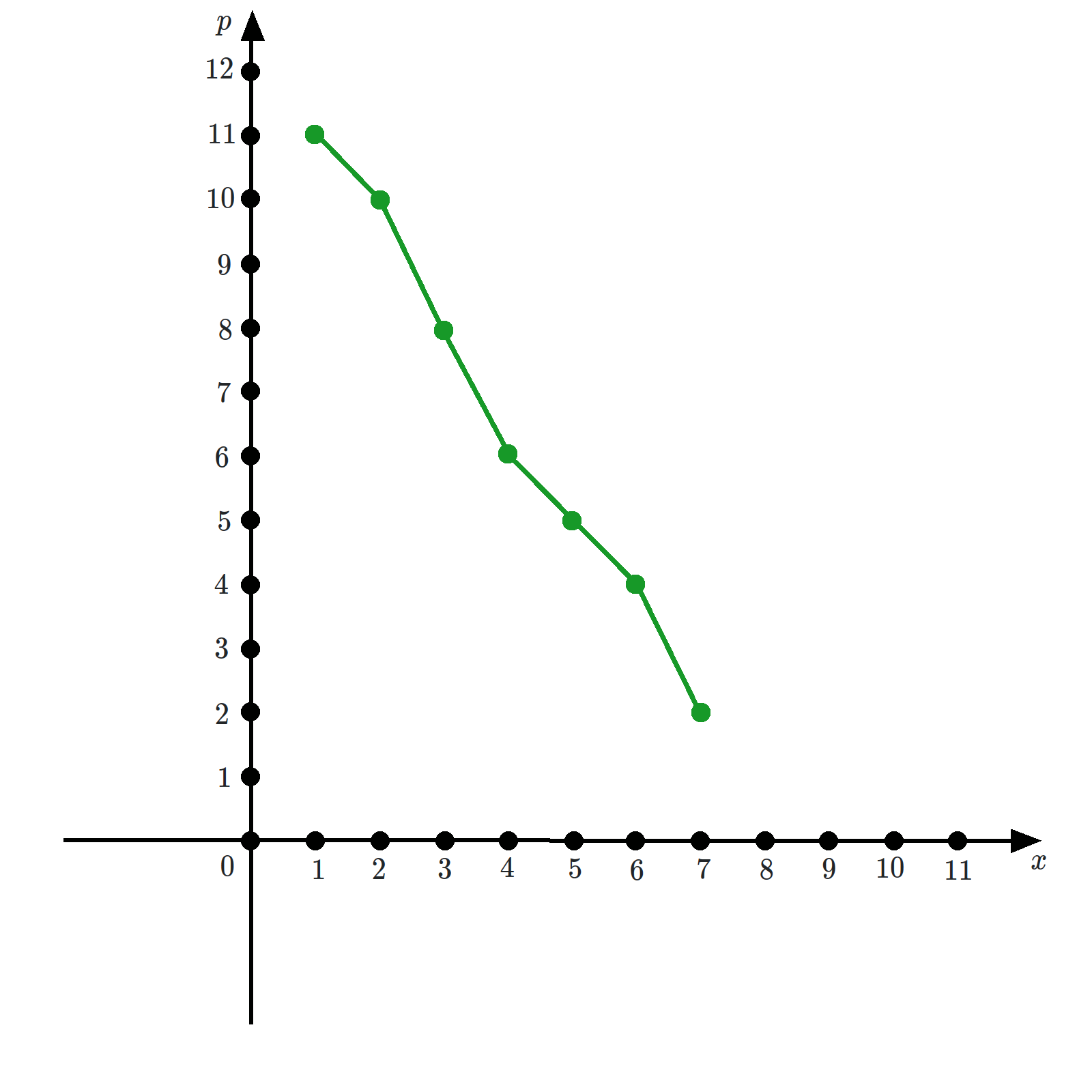
Demand is the function whose input is price \(p\) and output is the number of items that customers are willing to buy if the price is set to \(p\).
We will denote the demand function by \(\delta\).
Market Equilibrium
Let us now plot both the supply function and the demand function on the same graph.

When both curves are on the same graph, we (the store owners) can make the best decision on what should be the price of the frog. We will see that something bad happens if the price is too high. We will also see that another bad thing happens if the price is too low.
Let us first discuss an example in which we set the price too high: at \(\$8\). We will end up with \(5\) suppliers: Dali, van Gogh, Leonardo, Malevich, and Michelangelo. However, there are only \(3\) customers willing to pay this high price: Julius Caesar, Genghis Khan, and George Washington. We would end up with frogs that nobody wants to buy. That's bad. We learned our lesson: we shouldn't set the price too high.
We will now discuss another bad situation: setting the price too low: at \(\$5\). This time our store will be full of eager customers: Julius Caesar, Genghis Khan, George Washington, Winston Churchill, and Mao Zedong. That's 5 customers. However, we would have only 3 frogs (made by Dali, van Gogh, and Leonardo). Customer's won't be happy.
In order to satisfy all interested customers and not to have extra frogs, we need to pick the price \(p\) for which \(\sigma(p)=\delta(p)\). This price is called equilibrium price and is denoted by \(p_e\). The values \(\sigma(p_e)\) and \(\delta(p_e)\) are equal. They are denoted by \(x_e\). The ordered pair \((x_e,p_e)\) is called market equilibrium.
Producer surplus
We (the store owners) are not making money in this business. We are doing good for the world. We are taking the role of market makers. In reality market makers are making money. A lots of money. But we won't talk about that in this example.
Let's identify those who are making money. They are Salvador Dali, Vincent van Gogh, and Leonardo da Vinci. Salvador Dali was willing to sell the frog for \(\$2\). The price is \(\$6\). Salvador Dali may have some costs for making the frog, or he had better things to do and earn \(\$2\) in a more entertaining way. However, since he is receiving \(\$6\), he is really happy. He got \(\$4\) more than his minimal asking price. This \(\$4\) is called surplus. Vincent van Gogh has also a surplus of \(\$3\) and Leonardo da Vinci has a surplus of \(\$1\).
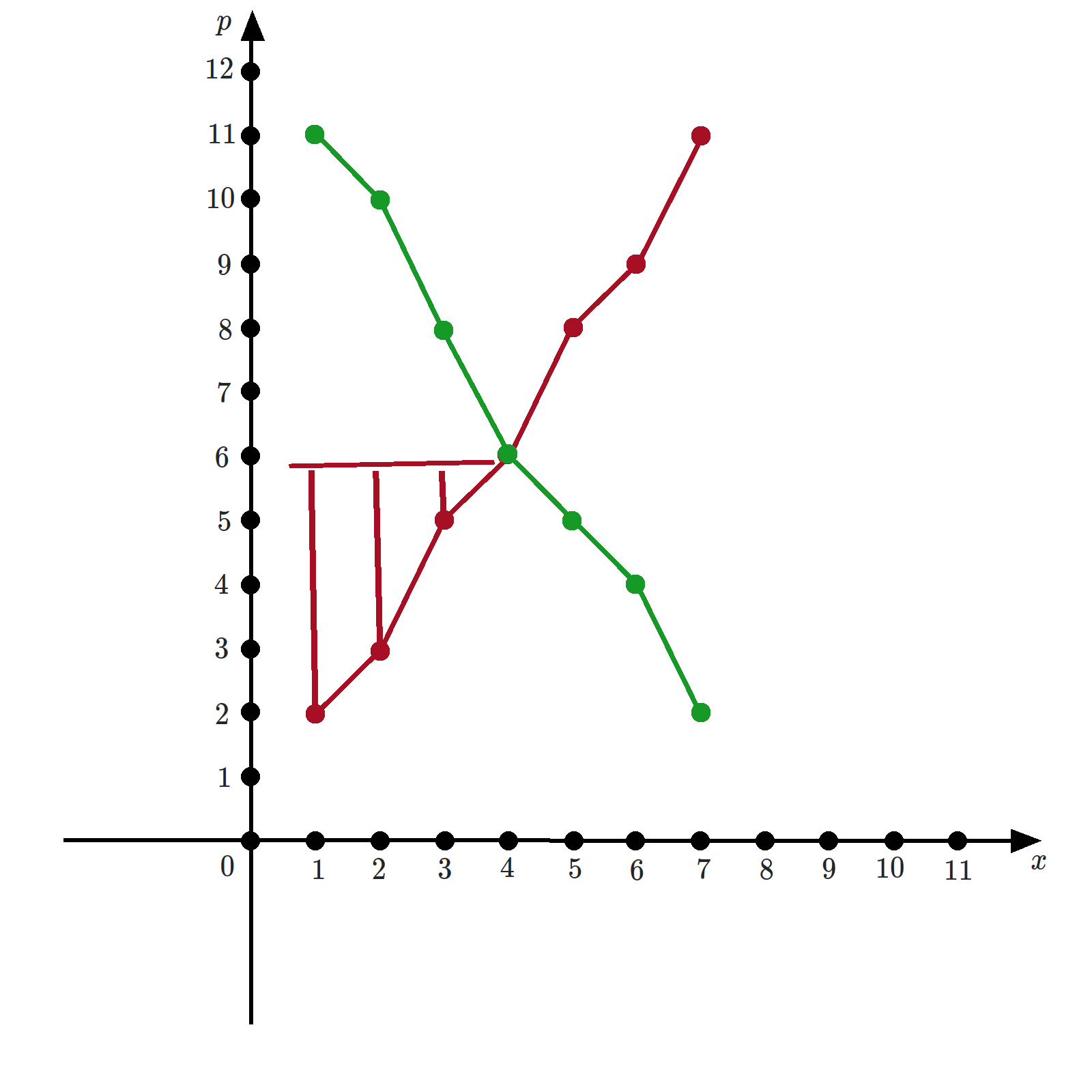
The surplus amounts of Salvador Dali, Vincent van Gogh, and Leonardo da Vinci are the lengths of the three vertical lines in the picture above. The sum of these numbers \(4+3+1=8\) is called producer surplus. This is the total amount of money that the artists make in the market equilibrium.
Consumer surplus
When the market is at equilibrium and the price of the frog is \(\$6\), then some consumers are also the winners. Julius Caesar was ready to pay \(\$11\) for a frog. Turns out he will be paying only \(\$6\). That means he will end up with change of \(\$5\). That \(\$5\) is his surplus. Genghis Khan will have a surplus of \(10-6=4\). George Washington will have a surplus of \(8-6=2\). The sum of all surpluses of all the tourists who are buying the frogs is \(6+4+2=12\). This sum is called consumer surplus.
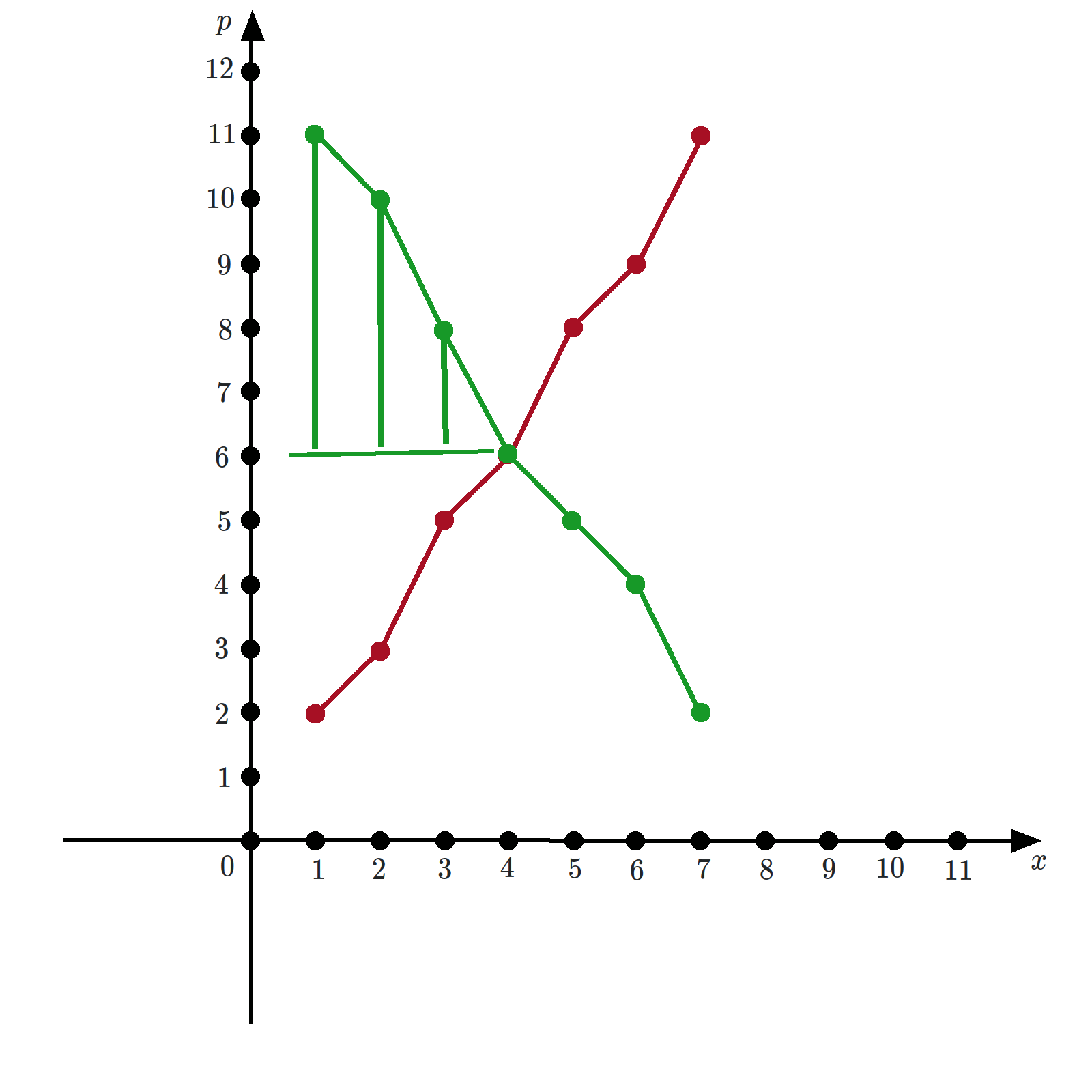
The surplus for each of the consumers is shown with a vertical line. The length of each line is the amount of the surplus that a given consumer has.
Continuous supply and demand functions
Now we will consider a more general case. The objects of trade are not frogs. The number of suppliers is not \(7\). The number of consumers is not \(7\). These numbers will not be even integers. We need to generalize the above concepts to situations where frogs are replaced with oil, gold, or salt.
In our frog selling business, the first frog could be produced for a price of \(\$2\). However, the second frog is more expensive. This concept generalizes in the following way. The first ounce (or gram if you are non-American) of salt can be obtained cheaply by suppliers who have a lot of salt. However, as the salt is taken away from the cheap suppliers, we are going to turn to the expensive ones. The cost of every subsequent gram (or ounce if you are American) is going to increase.
Similarly, the first gram of salt can be sold for very high price. We can find the customer who is willing to pay a lot. When this customer buys the salt, then the next amount of salt can't be sold for that much money. The price would go down.
As introduced earlier, the supplied function \(\sigma\) is defined in the following way. For a given price \(p\), \(\sigma(p)\) is the amount of items that can be obtained from the suppliers if the price is \(p\). Let us denote by \(S\) the inverse of \(\sigma\). This is the interpretation of \(S\). For given quantity \(x\), \(S(x)\) is the price that we would need to pay if we want our supply to reach the level \(x\).
The demand function \(\delta\) has its inverse \(D\). For given price \(p\), the number \(\delta(p)\) is the quantity of products that could be sold if the price is \(p\). For given quantity \(x\), the value \(D(x)\) is the price at which we will be selling the products if we want our sales to reach the level \(x\).
The market equilibrium is the ordered pair \((x_e,p_e)\) that satisfies \(x_e=\sigma(p_e)=\delta(p_e)\).
The conditions for the market equilibrium can be written in an equivalent form as \(S(x_e)=D(x_e)=p\).
The producer surplus and consumer surplus now become integrals instead of sums.
We will now solve a few exercises that involve the concepts defined above.